Teaching Math: Comparing Manipulatives

Often I am asked which manipulatives should I get? The manipulatives should suit the method. Jerry Mortensen repeatedly says, "All we do in math is count."

In MM we ask, "How many is.. four and two? We push the two blocks together and count the result. With the ridges on the blocks we can do this. With smooth rods you can't. Cuisinaire Rods, created by the Belgian primary school teacher Georges Cuisenaire (1891-1976) introduced in the 1950s are smooth and brightly colored but to do sums the child needs to know the number-color values.

In MM the emphasis is on counting and 'seeing' the numbers. What do you see when I say "six?" If you are doing subtraction, with Cuisinaire rods, the question has to be "what is the difference" between 2 and 4? Compare the rods. With MM combo blocks we would place the -2 on top of the 4 to cover up part of the four, and ask "How many do you see?" Two.

With smooth coloured rods (Cuisinaire) the child must learn the colours, and know that red is 2 and that 4 is purple. MM combo blocks are designed with ridges so the child can count how many a block is. The colours are not important in MM, other than to make the kit attractive. They are in fact Montessori colours because Jerry M was a Montessori teacher.

With Cuisinaire rods yellow is 5 units long and blue is 9. If the child has to learn the colours, she may hear, "How much is the blue bar added to the yellow?" Fourteen. But in art, she will learn that yellow and blue make green. However, in Cuisinaire rods, green is 3, not 14, an inconvenient truth for the math teacher. :) The point is that we should be making it easier for the child to discover math, not introducing another layer of code, in this case: colour, between the learner and the material. This is an obstacle to learning math, not an aide.

The Math-U-See blocks produced by Steve Demme are also Montessori colours and have ridges so the child can count how many without knowing the colors. The difference between MM and MUS, is to be found in the method.

In MM, our task as teachers is to decode this mathematical language, say, 13x12=156, into a spatial reality to assist understanding. The purpose of the manipulatives is to do just that. This is what that 'looks" like.

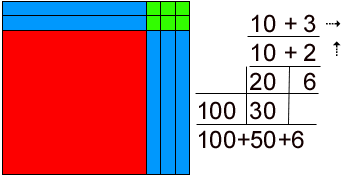
For a young child, could having a plastic block named 3 in her hand and counting 4 of them, then counting the unit square markings on the blocks and arriving at 12, be a relevant experience that might lead to understanding?

What use then is memorizing times tables?

re: plastic daisies and farm animals, sheep, etc. Having a child count plastic daisies is a mistake. That is not six she is looking at, it's a bunch of flowers. You can't teach area with flowers b/c you cannot push them into the corners, to then count the area. Similarly, stop using pizza to teach fractions. It is round and won't tesselate, so multiplication does not occur naturally as it does with rectangular blocks. Play with the farm animals, tell stories with them, but that's not the way to learn mathematics.

MORTENSON MORE THAN MATH employs manipulatives to enhance the child's ability to visualize math concepts, to decode the mathematical language into spatial reality.

The best way I know to explain the Mortensen Math system is to talk about memory first. How good is your short-term memory? More importantly, how good is your short-term memory with numbers? Suppose I gave you 12 numbers, each of them seven digits long. Do you think you could remember them for an hour? Five minutes? Do you think you could remember them long enough to write them down, even right after I told you?

Not likely. That's because you've been taught like everyone else to memorize the hard way. The hard way is how most students are being taught math today.

The truth is the entire math curriculum used in traditional teaching situations, employing textbooks, relies on memorizing nothing but FACTS, RULES, FORMULAE AND PROCESS!

Our job as educators is to decode this mathematical language of symbols into a concrete reality.

This is what the method does. Order your Teacher's Handbook (see panel at right) and start using Mortensen "more than math" right away!