Memorization and Understanding in Mathematics:
Teaching Math With Manipulatives

In this article I argue that mere memorization of symbols cannot logically lead to understanding, with reference to the thought expertiment: John Searle's Chinese room

To begin, here is a synopsis of the Chinese Room argument by John Searle:

from Wikipedia article:

"The Chinese room is a thought experiment by John Searle which first appeared in his paper "Minds, Brains, and Programs", published in Behavioral and Brain Sciences in 1980.[1] It addresses the question: if a machine can convincingly simulate an intelligent conversation, does it necessarily understand? In the experiment, Searle imagines himself in a room acting as a computer by manually executing a program that convincingly simulates the behavior of a native Chinese speaker. People outside the room slide Chinese characters under the door and Searle, to whom "Chinese writing is just so many meaningless squiggles",[1] is able to create sensible replies, in Chinese, by following the instructions of the program; that is, by moving papers around. The question arises whether Searle can be said to understand Chinese..?" ..Searle's Chinese Room Argument which holds that a program cannot give a computer a "mind" or "understanding", regardless of how intelligently it may make it behave.

He concludes that "I can have any formal program you like, ..but I still understand nothing."

The Chinese room is ..Searle's argument .. directed against functionalism and computationalism (philosophical positions inspired by AI), rather than the goals of applied AI research itself. [5] The argument leaves aside the question of creating an artificial mind by methods other than symbol manipulation."

Let's take Searle's thought experiment a bit further to see what it may tell us about children learning mathematics.

Suppose a dedicated human subject commits to translating Chinese into Russian in Cyrillic characters under the same conditions as John Searle in the above - she is a native speaker of English and knows nothing of either language She is given charts and manuals to assist her in producing the appropriate Russian response to given Chinese pictograms which she receives through a slot in the door. There are no pictures to aid her. She consults only with her books and charts and writes out the response on paper then slides it back out through the door. After a while she gets pretty good at this and has memorized certain combinations of Russian characters in response to Chinese pictograms that she has seen before. Later she is able to "translate" the Chinese symbols into Russian ones with only an occasional consultation with her Chinese - Russian dictionary and the grammar charts.

Can we say that she understands either language?

She still does not know what the Chinese pictograms mean in English, nor does she know the English equivalent of any Russian word. We might even provide her with tapes of how to pronounce each Chinese Pictogram and she can read the Chinese messages aloud so that a Chinese speaker could know what she is reading. Can she speak Chinese?

Let's say she has been translating a cookbook, remember no pictures, no smells or tastes either. Some of the recipes are among her favorite dishes but she has no way of knowing that. Would her mouth water in reading about them in Chinese the way it might if she read an English cookbook?

Serious contemplation of the experiment reveals that what is missing, what is so vital to our understanding of a language, even our own, is the relevant tactile motor kinaesthetic and other sensory experiences associated with each word or concept that denotes them. From birth we learn language cues in concert with the sight, feel, taste, smell or sound of an experience when the word is presented to us. The special case of Helen Keller is a vivid demonstration of how difficult learning language is without seeing, or hearing. Touch was the only major avenue for her to acquire language. Could she have achieved language at all if she also lacked hands? I argue, as does John Searle above, that meaning and understanding and the development of concepts are only possible with the relevant sensory experiences.

Given a string of symbols then, say "abhor," a child could not possibly impute any meaning to it, thus understanding is impossible merely by repetition, by memorization. Consider the jabberwocky poem. You can memorize it but it is still meaningless. Let's go a step further.

Given these symbols: x+5=14 a child could not tell if it was truthful or meaningless. By extension, memorizing 4x3=12 until the response "12" can be given to the prompt "4x3?" cannot be said to demonstrate understanding of multiplication, nor of 12 or 4 or 3 or anything at all.

Logically then, without the relevant sensory experiences, a child cannot come to understand multiplication or numbers merely by memorizing times tables.

What might the relevant experiences be?

For a young child, could having a plastic block named 3 in her hand and counting 4 of them, then counting the unit square markings on the blocks and arriving at 12, be a relevant experience that might lead to understanding?

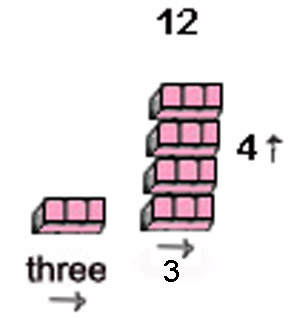
What use then, is memorizing times tables?

MORTENSON MORE THAN MATH employs manipulatives to enhance the child's ability to visualize math concepts, to decode the mathematical language into spatial reality.

The best way I know to explain the Mortensen Math system is to talk about memory first. How good is your short-term memory? More importantly, how good is your short-term memory with numbers? Suppose I gave you 12 numbers, each of them seven digits long. Do you think you could remember them for an hour? Five minutes? Do you think you could remember them long enough to write them down, even right after I told you?

Not likely. That's because you've been taught like everyone else to memorize the hard way. The hard way is how most students are taught math as well.

The truth is the entire math curriculum used in traditional teaching situations, employing textbooks, relies on memorizing nothing but FACTS, RULES, FORMULAE AND PROCESS!

Our job as educators is to decode this mathematical language of symbols into a concrete reality. This is what the method does.