Teaching Math With Manipulatives

How does a thing acquire meaning?

How does y = Mx+C acquire meaning?

How does "the square on the hypotenuse equals the sum of the squares on the other two sides" acquire meaning?

How does "6" become meaningful?

One of my lifelong philosophical investigations has been the acquisition of meaning. There is a TV show that ends its credits with a boy pointing at a tree on a hillside and asking, "What does that mean?" Is that a sensible question? Consider if the tree has meaning, if the tree on a hillside has a meaning, if a tree on a hillside against a cloudless sky has meaning. I think what this line of questioning reveals is that we look for patterns of relationships to elicit meaning. Humans are essentially pattern recognizers. I wrote an undergraduate thesis entitled, "Intelligence as the ability to recognize, extend and create patterns." In it I wrote that a pattern is a relationship between at least two things. For there to be a pattern there must be at least two things. In the case of a dot on an infinite field there is no pattern. But a dot on a field with finite boundaries must have a relation to at least the boundary, or in the more pedestrian case, the dot has a relationship with the edge of the paper. By extrapolation, an isolated event, a 'random' event is not a pattern. For an event to have a pattern there must at least exist a context for the event. If there are two such events, then of course, we have a pattern.

One way to investigate the acquisition of meaning is to consider learning a foreign language as an adult. I have taught ESL. One typically begins with some vocabulary, some examples of familiar objects: dog, cat, man, car. This is just re-naming. The meaning for the learner is still the original word/object relationship they discovered growing up. My understanding of cat is not enhanced by learning that, in French, it is called "chat."

In some languages I am told, there are concepts that do not exist in other languages. If a student should acquire the new concept in the new (for her) language as an adult, then she would be able to tell us something about the acquisition of meaning. Without her we have to introspect a bit more. If I learn a new word in a foreign language, say, in a vocabulary list, such as perro, broma, barato, etc. I may memorize the list and pair them with the equivalent words in my first language but it is a chore and I need many reviews, even then I have to translate the word to English to assign meaning to the object to which the word refers. I think what is missing to give the new Spanish word meaning for me is having the relevant experiences with the object that a native speaker might have when acquiring knowledge of the object in the quotidian way. This is to say that there are schemas (pre-concepts) and actual physical experiences (sensory experiences) that someone would have in association with learning a word. Take "perro" for example. When a Spanish child first meets a perro his parents might use the word as it licks his face and that experience is what the child recalls when he hears the word subsequently. Also, when he experiences the face-licking again he might utter the word "perro." Importantly, there will be feelings associated with the use of the word, and vice versa, the word can elicit the feelings.

This is an important feature of meaning acquisition. To put it bluntly, for there to be meaning, there must be feeling. Could there be a rote recall of an equivalent meaning as in vocabulary lists? Yes, of course, but for there to be a visceral understanding of a word or concept, there must be a feeling associated with it.

Therefore when teaching, we must seek to evoke feelings in association with a concept in order to ensure that students acquire meaning viscerally.

A corollary of this is, that if there are no feelings associated with an experience then it is meaningless. An example of this may be when a student witnesses an experiment in science, chemistry, say, perhaps litmus paper turning colour when in contact with an acid or akali, and having no accompanying feeling, she simply shrugs and says, "So what?" In this case I am prepared to admit that that is an appropriate response and that we, as educators, ought to respond positively to it by changing our approach, rather than, say, reproaching the student for having a bad attitude.

Again, to acquire meaning requires that there be feelings present. What kind of feelings? There is an amazing range of possible feelings that could be associated with an experience. Consider dissecting a frog. You can easily imagine the range of feelings this could engender, ickiness, amazement, wonder, fear, disgust, and so on. No doubt all of us remember that experiment vividly. For this query, it is not the one, intended, scientific feeling that I am concerned with. I wish only to observe that with strong feelings comes strong learning. Focusing the learning experience is another project. Thus, in the case of learning math, where meaning is often lacking and the learning is sometimes considered tedious, it is obvious that we must strive to present the opportunity for the students to experience feelings when learning math. One feeling we all would like them to experience is the joy that comes with success.

How shall this be done?

One way is to employ the "explore and discover" principle. In practice this looks a lot like play, but it is directed play.

Exploring and discovering are activities naturally satisfying curiosity. And like attaining understanding, directed play, or exploring and discovering, produces good feelings.

Understanding a concept is usually accompanied by the production of dopamine and endorphins in the brain, in short, pleasure. When that little light goes on, and you finally "get it," it feels good!

Jean Piaget and Maria Montessori have shown that understanding of concepts occurs naturally in children if the appropriate sensori-motor experiences can be had. That means the necessary opportunity for exploring and discovering concepts must be provided for the children to learn naturally; in other words, play.

But not just any play, if you want children to learn language you must provide a language-rich environment. If you want children to learn math concepts you must provide a math-rich environment. More on that later, let's get this straight: sensori-motor experiences of the right kind are necessary for the acquisition of schemas - groups of experiences, that can be assimilated and synthesized into concepts or accommodated by the child's mind. This sense of understanding is enjoyable and is all that is needed for successful learning.

It is our mission as teachers and parents to provide the appropriate materials and situations for this to happen, and sometimes just to get out of the way.

Story Telling is another way to impart meaning to a learning experience, particularly if the story-telling evokes feelings. The best teachers make the story dramatic.

While that made me happy, by 1998 the demand on my schedule had become so heavy I knew had to find another way to reach more people. My solution was to record my workshop on video. I willingly sent the tapes to interested parties I couldn't teach in person. The problem with that scheduling solution was no one could ask questions. Extra explanations were missing because I wasn't there in person at the white board. That led me to the development of a handbook.

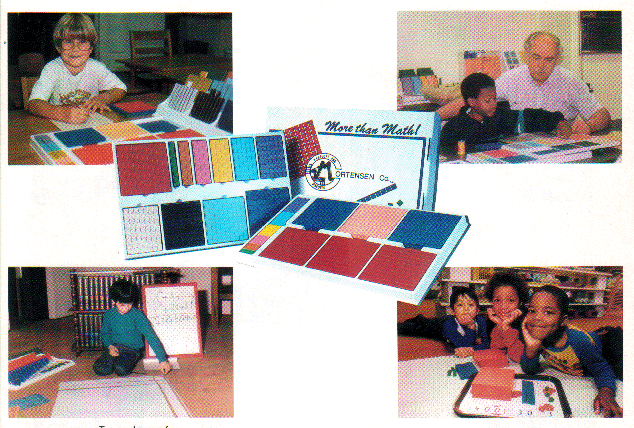
I'll tell you more about that in a minute. You probably want to know more about the Mortensen Math system first.

MORTENSON MORE THAN MATH employs manipulatives to enhance the child's ability to visualize math concepts, to decode the mathematical language into spatial reality.

The best way I know to explain the Mortensen Math system is to talk about memory first. How good is your short-term memory? More importantly, how good is your short-term memory with numbers? Suppose I gave you 12 numbers, each of them seven digits long. Do you think you could remember them for an hour? Five minutes? Do you think you could remember them long enough to write them down, even right after I told you?

Not likely. That's because you've been taught like everyone else to memorize the hard way. The hard way is how most students are taught math as well.

The truth is the entire math curriculum used in traditional teaching situations, employing textbooks, relies on memorizing nothing but FACTS, RULES, FORMULAE AND PROCESS!

Our job as educators is to decode this mathematical language of symbols into a concrete reality. This is what the method does. The Complete Method, $25, available in eBook from Amazon